Give the definition of an infinitely large function at a point. Limit of a Function - MT1205: Mathematical Analysis for Economists - Business Informatics. Proof of the theorem on the product of a bounded function and an infinitesimal one

Function y=f(x) called infinitesimal at x→a or when x→∞, if or , i.e. an infinitesimal function is a function whose limit at a given point is zero.

Examples.

1. Function f(x)=(x-1) 2 is infinitesimal at x→1, since (see figure).

2. Function f(x)= tg x– infinitesimal at x→0.

3. f(x)= log(1+ x) – infinitesimal at x→0.

4. f(x) = 1/x– infinitesimal at x→∞.

Let us establish the following important relationship:

Theorem. If the function y=f(x) representable with x→a as a sum of a constant number b and infinitesimal magnitude α(x): f (x)=b+ α(x) That .

Conversely, if , then f (x)=b+α(x), Where a(x)– infinitesimal at x→a.

Proof.

1. Let us prove the first part of the statement. From equality f(x)=b+α(x) should |f(x) – b|=| α|. But since a(x) is infinitesimal, then for arbitrary ε there is δ – a neighborhood of the point a, in front of everyone x from which, values a(x) satisfy the relation |α(x)|< ε. Then |f(x) – b|< ε. And this means that.

2. If , then for any ε >0 for all X from some δ – neighborhood of a point a will |f(x) – b|< ε. But if we denote f(x) – b= α, That |α(x)|< ε, which means that a– infinitesimal.

Let's consider the basic properties of infinitesimal functions.

Theorem 1. The algebraic sum of two, three, and in general any finite number of infinitesimals is an infinitesimal function.

Proof. Let us give a proof for two terms. Let f(x)=α(x)+β(x), where and . We need to prove that for any arbitrary small ε > 0 found δ> 0, such that for x, satisfying the inequality |x – a|<δ , performed |f(x)|< ε.

So, let’s fix an arbitrary number ε > 0. Since according to the conditions of the theorem α(x) is an infinitesimal function, then there is such δ 1 > 0, which is |x – a|< δ 1 we have |α(x)|< ε / 2. Likewise, since β(x) is infinitesimal, then there is such δ 2 > 0, which is |x – a|< δ 2 we have | β(x)|< ε / 2.

Let's take δ=min(δ 1 , δ2 } .Then in the vicinity of the point a radius δ each of the inequalities will be satisfied |α(x)|< ε / 2 and | β(x)|< ε / 2. Therefore, in this neighborhood there will be

|f(x)|=| α(x)+β(x)| ≤ |α(x)| + | β(x)|< ε /2 + ε /2= ε,

those. |f(x)|< ε, which is what needed to be proved.

Theorem 2. Product of an infinitesimal function a(x) for a limited function f(x) at x→a(or when x→∞) is an infinitesimal function.

Proof. Since the function f(x) is limited, then there is a number M such that for all values x from some neighborhood of a point a|f(x)|≤M. Moreover, since a(x) is an infinitesimal function at x→a, then for an arbitrary ε > 0 there is a neighborhood of the point a, in which the inequality will hold |α(x)|< ε /M. Then in the smaller of these neighborhoods we have | αf|< ε /M= ε. And this means that af– infinitesimal. For the occasion x→∞ the proof is carried out similarly.

From the proven theorem it follows:

Corollary 1. If and, then.

Corollary 2. If c= const, then .

Theorem 3. Ratio of an infinitesimal function α(x) per function f(x), the limit of which is different from zero, is an infinitesimal function.

Proof. Let . Then 1 /f(x) there is a limited function. Therefore, a fraction is the product of an infinitesimal function and a limited function, i.e. function is infinitesimal.

The function is called infinitesimal at
or when
, If
or
.

For example: function
infinitesimal at
;
infinitesimal at
.

function Note 1.
Without indicating the direction of change of the argument, no function can be called infinitesimal. Yes, the function
at
is infinitesimal, and when
).

it is no longer infinitesimal ( Note 2.
From the definition of the limit of a function at a point, for infinitesimal functions the following inequality holds:

We will use this fact more than once in the future. Let's establish some important

properties of infinitesimal functions. Theorem
(about the connection between a function, its limit and the infinitesimal): If the function can be represented as a sum of a constant number A
Without indicating the direction of change of the argument, no function can be called infinitesimal. Yes, the function
and infinitesimal function

, then the number

Proof:
.

From the conditions of the theorem it follows that the function
:
Let's express from here
. Since the function
infinitesimal, the inequality holds for it
, then for the expression (

) the inequality also holds
.

properties of infinitesimal functions. And this means that
(reverse): if
, then the function can be represented as a sum of a constant number can be represented as the sum of a number
and infinitesimal at
functions
.

, then the number

, i.e.
Because
, then for
inequality holds
(*) Consider the function

as a single one and rewrite inequality (*) in the form
From the last inequality it follows that the value (
) is infinitesimal at
.

. Let's denote it
Where

. The theorem has been proven. Theorem 1

, then the number

. The algebraic sum of a finite number of infinitesimal functions is an infinitesimal function.

Let us carry out the proof for two terms, since for any finite number of terms it is given in a similar way.
Let
And
infinitesimal at
functions and
– the sum of these functions. Let us prove that for
, there is such a thing X that's for everyone
, satisfying the inequality
.

Since the function
infinitesimal function
, there is such a thing
, then for
.

Since the function
infinitesimal function
, and therefore there is such , there is such a thing
, then for
.

Let's take equal to the smaller number Let , then in –neighborhood of the point A the inequalities will be satisfied
,
.

Let's create a function module
and evaluate its significance.

That is
, then the function is infinitesimal, which is what needed to be proven.

Theorem 2. Product of an infinitesimal function
Without indicating the direction of change of the argument, no function can be called infinitesimal. Yes, the function
for a limited function
is an infinitesimal function.

, then the number

Since the function
bounded, then there is a positive number
, there is such a thing , then for
.

Since the function
infinitesimal at
, then there is such –neighborhood of a point , there is such a thing in this neighborhood the inequality
.

Consider the function
and evaluate its module

So
, and then
– infinitesimal.

The theorem has been proven.

Limit theorems.

Theorem 1. The limit of an algebraic sum of a finite number of functions is equal to the algebraic sum of the limits of these functions

, then the number

To prove it, it is enough to consider two functions; this will not violate the generality of reasoning.

Let us carry out the proof for two terms, since for any finite number of terms it is given in a similar way.
,
.

According to the theorem about the connection between a function, its limit and an infinitesimal function
Let
can be represented in the form
Where
Let
– infinitesimal at
.

Let's find the sum of functions
Let

Magnitude
there is a constant value
– the quantity is infinitesimal. So the function
presented as the sum of a constant value and an infinitesimal function.

Then the number
is the limit of the function
functions

The theorem has been proven.

Theorem 2 . The limit of the product of a finite number of functions is equal to the product of the limits of these functions

, then the number

Without losing the generality of reasoning, we will carry out a proof for two functions
Let
.

Let it be then
,

Let's find the product of functions
Let

Magnitude
there is a constant quantity, an infinitesimal function. Therefore, the number
is the limit of the function
, that is, the equality is true

Consequence:
.

Theorem 3. The limit of the quotient of two functions is equal to the quotient of the limits of these functions if the limit of the denominator is nonzero

.

Proof: Let
,

Then
,
.

Let's find the quotient and perform some identical transformations on it

Magnitude constant, fraction
infinitely small. Therefore, the function represented as the sum of a constant number and an infinitesimal function.

Then
.

Comment. Theorems 1–3 have been proven for the case
. However, they may be applicable when
, since the proof of the theorems in this case is carried out similarly.

For example. Find limits:

The first and second are wonderful limits.

Function not defined at
. However, its values ​​in the vicinity of the zero point exist. Therefore, we can consider the limit of this function at
. This limit is called first wonderful limit .

It looks like:
.

For example . Find limits: 1.
. Designate
, If
, That
.
; 2.
. Let us transform this expression so that the limit is reduced to the first remarkable limit.
; 3..

Let's consider a variable of the form
, wherein takes the values ​​of natural numbers in ascending order. Let's give different meanings: if

Giving the following values ​​from the set
, it is easy to see that the expression
Without indicating the direction of change of the argument, no function can be called infinitesimal. Yes, the function
will
. Moreover, it is proven that
has a limit. This limit is indicated by the letter :
.

Number irrational:
.

Now consider the limit of the function
Without indicating the direction of change of the argument, no function can be called infinitesimal. Yes, the function
. This limit is called second remarkable limit

It looks like
.

For example.

A)
. Expression
replace it with the product identical factors
, we apply the product limit theorem and the second remarkable limit;
b)
. Let's put
,
.

, Then The second remarkable limit is used in

continuous compounding problem

,

When calculating cash income on deposits, they often use the compound interest formula, which looks like: Where

- initial contribution,

- annual bank interest,

- number of interest accruals per year,

- time, in years.

.

However, in theoretical studies, when justifying investment decisions, they often use the formula of the exponential (exponential) growth law

The formula for the exponential growth law is obtained as a result of applying the second remarkable limit to the compound interest formula

Consider the function
Continuity of functions. defined at some point and some neighborhood of the point
.

. Let the function have the value at the indicated point
Definition 1. Function called continuous at a point
.

, if it is defined in a neighborhood of a point, including the point itself and

The definition of continuity can be formulated differently.
Let the function ,
defined at some value . If the argument
give an increment

, then the function will receive an increment Let the function at the point

continuous (by the first definition of continuity of a function at a point), That is, if the function is continuous at the point
, then an infinitesimal increment of the argument

at this point there corresponds an infinitesimal increment of the function.

The converse is also true: if an infinitesimal increment in the argument corresponds to an infinitesimal increment in the function, then the function is continuous.
Definition 2. Function
is called continuous at (at point
.

), if it is defined at this point and some of its surroundings and if

Taking into account the first and second definitions of the continuity of a function at a point, we can obtain the following statement:
or
. Let's put
.

, But
Therefore, in order to find the limit of a continuous function at it is enough to use an analytical function expression instead of an argument .

substitute its value Definition 3. A function that is continuous at every point of a certain region is called continuous

in this area.

For example:
is continuous at all points of the domain of definition.

Let's use the second definition of continuity of a function at a point. To do this, take any value of the argument and give it an increment
. Let's find the corresponding increment of the function

Example 2. Prove that the function
continuous at all points from
.

Let's give the argument increment
, then the function will be incremented

Let's find since the function
, that is, limited.

Similarly, it can be proven that all basic elementary functions are continuous at all points of their domain of definition, that is, the domain of definition of an elementary function coincides with its domain of continuity.

Definition 4. If the function
continuous at every point of some interval
, then we say that the function is continuous on this interval.

Calculus of infinitesimals and larges

Infinitesimal calculus- calculations performed with infinitesimal quantities, in which the derived result is considered as an infinite sum of infinitesimals. The calculus of infinitesimals is a general concept for differential and integral calculus, which forms the basis of modern higher mathematics. The concept of an infinitesimal quantity is closely related to the concept of limit.

Infinitesimal

Subsequence a n called infinitesimal, If . For example, a sequence of numbers is infinitesimal.

The function is called infinitesimal in the vicinity of a point x 0 if .

The function is called infinitesimal at infinity, If or .

Also infinitesimal is a function that is the difference between a function and its limit, that is, if , That f(x) − a = α( x) , .

Infinitely large quantity

Subsequence a n called infinitely large, If .

The function is called infinitely large in the vicinity of a point x 0 if .

The function is called infinitely large at infinity, If or .

In all cases, infinity to the right of equality is implied to have a certain sign (either “plus” or “minus”). That is, for example, the function x sin x is not infinitely large at .

Properties of infinitely small and infinitely large

Comparison of infinitesimal quantities

How to compare infinitesimal quantities?
The ratio of infinitesimal quantities forms the so-called uncertainty.

Definitions

Suppose we have infinitesimal values ​​α( x) and β( x) (or, which is not important for the definition, infinitesimal sequences).

To calculate such limits it is convenient to use L'Hopital's rule.

Comparison examples

Using ABOUT-symbolism, the results obtained can be written in the following form x 5 = o(x 3). In this case, the following entries are true: 2x 2 + 6x = O(x) And x = O(2x 2 + 6x).

Equivalent values

Definition

If , then the infinitesimal quantities α and β are called equivalent ().
It is obvious that equivalent quantities are a special case of infinitesimal quantities of the same order of smallness.

When the following equivalence relations are valid: , , .

properties of infinitesimal functions.

The limit of the quotient (ratio) of two infinitesimal quantities will not change if one of them (or both) is replaced by an equivalent quantity.

This theorem has practical significance when finding limits (see example).

Usage example

Replacing sin 2x equivalent value 2 x, we get

Historical sketch

The concept of “infinitesimal” was discussed back in ancient times in connection with the concept of indivisible atoms, but was not included in classical mathematics. It was revived again with the advent of the “method of indivisibles” in the 16th century - dividing the figure under study into infinitesimal sections.

In the 17th century, the algebraization of infinitesimal calculus took place. They began to be defined as numerical quantities that are less than any finite (non-zero) quantity and yet not equal to zero. The art of analysis consisted in drawing up a relation containing infinitesimals (differentials) and then integrating it.

Old school mathematicians put the concept to the test infinitesimal harsh criticism. Michel Rolle wrote that the new calculus is “ set of ingenious mistakes"; Voltaire caustically remarked that calculus is the art of calculating and accurately measuring things whose existence cannot be proven. Even Huygens admitted that he did not understand the meaning of differentials of higher orders.

As an irony of fate, one can consider the emergence in the middle of the century of non-standard analysis, which proved that the original point of view - actual infinitesimals - was also consistent and could be used as the basis for analysis.

see also

Wikimedia Foundation.

2010.

See what “Infinitely large” is in other dictionaries: The variable quantity Y is the inverse of the infinitesimal quantity X, that is, Y = 1/X...

Big Encyclopedic Dictionary The variable y is the inverse of the infinitesimal x, that is, y = 1/x. * * * INFINITELY LARGE INFINITELY LARGE, variable quantity Y, inverse to the infinitesimal quantity X, that is, Y = 1/X ...

encyclopedic Dictionary In mathematics, a variable quantity that, in a given process of change, becomes and remains greater in absolute value than any predetermined number. Study of B. b. quantities can be reduced to the study of infinitesimals (See... ...

Great Soviet Encyclopedia

Infinitesimal functions infinitesimal(b.m.) with %%x \to a \in \overline(\mathbb(R))%%, if with this tendency of the argument the limit of the function is equal to zero.

The concept of b.m. function is inextricably linked with instructions to change its argument. We can talk about b.m. functions at %%a \to a + 0%% and at %%a \to a - 0%%. Usually b.m. functions are denoted by the first letters of the Greek alphabet %%\alpha, \beta, \gamma, \ldots%%

Examples

1. The function %%f(x) = x%% is b.m. at %%x \to 0%%, since its limit at the point %%a = 0%% is zero. According to the theorem about the connection between the two-sided limit and the one-sided limit, this function is b.m. both with %%x \to +0%% and with %%x \to -0%%.
2. Function %%f(x) = 1/(x^2)%% - b.m. at %%x \to \infty%% (as well as at %%x \to +\infty%% and at %%x \to -\infty%%).

A constant number other than zero, no matter how small in absolute value, is not a b.m. function.

For constant numbers, the only exception is zero, since the function %%f(x) \equiv 0%% has a zero limit.

Theorem

The function %%f(x)%% has at the point %%a \in \overline(\mathbb(R))%% of the extended number line a final limit equal to the number %%b%% if and only if this function equal to the sum of this number %%b%% and b.m. functions %%\alpha(x)%% with %%x \to a%%, or $$ \exists~\lim\limits_(x \to a)(f(x)) = b \in \mathbb(R ) \Leftrightarrow \left(f(x) = b + \alpha(x)\right) \land \left(\lim\limits_(x \to a)(\alpha(x) = 0)\right). $$

Properties of infinitesimal functions

1. According to the rules of passage to the limit with %%c_k = 1~ \forall k = \overline(1, m), m \in \mathbb(N)%%, the following statements follow:
2. The sum of the final number of b.m. functions for %%x \to a%% is b.m. at %%x \to a%%.

The product of any number b.m. functions for %%x \to a%% is b.m. at %%x \to a%%.

Product b.m. functions at %%x \to a%% and a function bounded in some punctured neighborhood %%\stackrel(\circ)(\text(U))(a)%% of point a, there is b.m. at %%x \to a%% function.

It is clear that the product of a constant function and b.m. at %%x \to a%% there is b.m. function at %%x \to a%%.

Equivalent infinitesimal functions equivalent Infinitesimal functions %%\alpha(x), \beta(x)%% for %%x \to a%% are called

and write %%\alpha(x) \sim \beta(x)%%, if

$$ \lim\limits_(x \to a)(\frac(\alpha(x))(\beta(x))) = \lim\limits_(x \to a)(\frac(\beta(x) )(\alpha(x))) = 1. $$

Let %%\alpha(x), \alpha_1(x), \beta(x), \beta_1(x)%% be b.m. functions for %%x \to a%%, and %%\alpha(x) \sim \alpha_1(x); \beta(x) \sim \beta_1(x)%%, then $$ \lim\limits_(x \to a)(\frac(\alpha(x))(\beta(x))) = \lim\ limits_(x \to a)(\frac(\alpha_1(x))(\beta_1(x))). $$

Equivalent b.m. functions.

Let %%\alpha(x)%% be b.m. function at %%x \to a%%, then

1. %%\sin(\alpha(x)) \sim \alpha(x)%%
2. %%\displaystyle 1 - \cos(\alpha(x)) \sim \frac(\alpha^2(x))(2)%%
3. %%\tan \alpha(x) \sim \alpha(x)%%
4. %%\arcsin\alpha(x) \sim \alpha(x)%%
5. %%\arctan\alpha(x) \sim \alpha(x)%%
6. %%\ln(1 + \alpha(x)) \sim \alpha(x)%%
7. %%\displaystyle\sqrt[n](1 + \alpha(x)) - 1 \sim \frac(\alpha(x))(n)%%
8. %%\displaystyle a^(\alpha(x)) - 1 \sim \alpha(x) \ln(a)%%

Example

$$ \begin(array)(ll) \lim\limits_(x \to 0)( \frac(\ln\cos x)(\sqrt(1 + x^2) - 1)) & = \lim\limits_ (x \to 0)(\frac(\ln(1 + (\cos x - 1)))(\frac(x^2)(4))) = \\ & = \lim\limits_(x \to 0)(\frac(4(\cos x - 1))(x^2)) = \\ & = \lim\limits_(x \to 0)(-\frac(4 x^2)(2 x^ 2)) = -2 \end(array) $$

Infinitely large functions

Infinitesimal functions infinitely large(b.b.) with %%x \to a \in \overline(\mathbb(R))%%, if with this tendency of the argument the function has an infinite limit.

Similar to b.m. functions concept b.b. function is inextricably linked with instructions to change its argument. We can talk about b.b. functions with %%x \to a + 0%% and %%x \to a - 0%%. The term “infinitely large” does not speak about the absolute value of the function, but about the nature of its change in the vicinity of the point in question. No constant number, no matter how large in absolute value, is infinitely large.

Examples

1. Function %%f(x) = 1/x%% - b.b. at %%x \to 0%%.
2. Function %%f(x) = x%% - b.b. at %%x \to \infty%%.

If the definition conditions $$ \begin(array)(l) \lim\limits_(x \to a)(f(x)) = +\infty, \\ \lim\limits_(x \to a)(f( x)) = -\infty, \end(array) $$

then they talk about positive or negative b.b. at %%a%% function.

Example

Function %%1/(x^2)%% - positive b.b. at %%x \to 0%%.

The connection between b.b. and b.m. functions

If %%f(x)%% is b.b. with %%x \to a%% function, then %%1/f(x)%% - b.m.

at %%x \to a%%. If %%\alpha(x)%% - b.m. for %%x \to a%% is a non-zero function in some punctured neighborhood of the point %%a%%, then %%1/\alpha(x)%% is b.b. at %%x \to a%%.

Properties of infinitely large functions

Let us present several properties of the b.b. functions. These properties follow directly from the definition of b.b. functions and properties of functions having finite limits, as well as from the theorem on the connection between b.b. and b.m. functions.

1. The product of a finite number of b.b. functions for %%x \to a%% is b.b. function at %%x \to a%%. Indeed, if %%f_k(x), k = \overline(1, n)%% - b.b. function at %%x \to a%%, then in some punctured neighborhood of the point %%a%% %%f_k(x) \ne 0%%, and by the connection theorem b.b. and b.m. functions %%1/f_k(x)%% - b.m. function at %%x \to a%%. It turns out %%\displaystyle\prod^(n)_(k = 1) 1/f_k(x)%% - b.m function for %%x \to a%%, and %%\displaystyle\prod^(n )_(k = 1)f_k(x)%% - b.b. function at %%x \to a%%.
2. Product b.b. functions for %%x \to a%% and a function which in some punctured neighborhood of the point %%a%% in absolute value is greater than a positive constant is b.b. function at %%x \to a%%. In particular, the product b.b. a function with %%x \to a%% and a function that has a finite non-zero limit at the point %%a%% will be b.b. function at %%x \to a%%.

The sum of a function bounded in some punctured neighborhood of the point %%a%% and b.b. functions with %%x \to a%% is b.b. function at %%x \to a%%.

For example, the functions %%x - \sin x%% and %%x + \cos x%% are b.b. at %%x \to \infty%%.

3. The sum of two b.b. functions at %%x \to a%% there is uncertainty. Depending on the sign of the terms, the nature of the change in such a sum can be very different.

   Example

   Let the functions %%f(x)= x, g(x) = 2x, h(x) = -x, v(x) = x + \sin x%% be given. functions at %%x \to \infty%%. Then:

   • %%f(x) + g(x) = 3x%% - b.b. function at %%x \to \infty%%;
   • %%f(x) + h(x) = 0%% - b.m. function at %%x \to \infty%%;
   • %%h(x) + v(x) = \sin x%% has no limit at %%x \to \infty%%.

INFINITESMALL FUNCTIONS AND THEIR BASIC PROPERTIES

Function y=f(x) called infinitesimal at x→a or when x→∞, if or , i.e. an infinitesimal function is a function whose limit at a given point is zero.

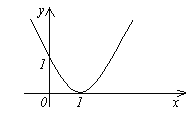
Examples.

Let us establish the following important relationship:

Theorem. If the function y=f(x) representable with x→a as a sum of a constant number b and infinitesimal magnitude α(x): f (x)=b+ α(x) That .

Conversely, if , then f (x)=b+α(x), Where a(x)– infinitesimal at x→a.

Proof.

Let's consider the basic properties of infinitesimal functions.

Theorem 1. The algebraic sum of two, three, and in general any finite number of infinitesimals is an infinitesimal function.

Proof. Let us give a proof for two terms. Let f(x)=α(x)+β(x), where and . We need to prove that for any arbitrary small ε > 0 found δ> 0, such that for x, satisfying the inequality |x – a|<δ , performed |f(x)|< ε.

So, let’s fix an arbitrary number ε > 0. Since according to the conditions of the theorem α(x) is an infinitesimal function, then there is such δ 1 > 0, which is |x – a|< δ 1 we have |α(x)|< ε / 2. Likewise, since β(x) is infinitesimal, then there is such δ 2 > 0, which is |x – a|< δ 2 we have | β(x)|< ε / 2.

Let's take δ=min(δ 1 , δ2 } .Then in the vicinity of the point a radius δ each of the inequalities will be satisfied |α(x)|< ε / 2 and | β(x)|< ε / 2. Therefore, in this neighborhood there will be

|f(x)|=| α(x)+β(x)| ≤ |α(x)| + | β(x)|< ε /2 + ε /2= ε,

those. |f(x)|< ε, which is what needed to be proved.

Theorem 2. Product of an infinitesimal function a(x) for a limited function f(x) at x→a(or when x→∞) is an infinitesimal function.

Proof. Since the function f(x) is limited, then there is a number M such that for all values x from some neighborhood of a point a|f(x)|≤M. Moreover, since a(x) is an infinitesimal function at x→a, then for an arbitrary ε > 0 there is a neighborhood of the point a, in which the inequality will hold |α(x)|< ε /M. Then in the smaller of these neighborhoods we have | αf|< ε /M= ε. And this means that af– infinitesimal. For the occasion x→∞ the proof is carried out similarly.

From the proven theorem it follows:

Corollary 1. If and, then.

Corollary 2. If c= const, then .

Theorem 3. Ratio of an infinitesimal function α(x) per function f(x), the limit of which is different from zero, is an infinitesimal function.

Proof. Let . Then 1 /f(x) there is a limited function. Therefore the fraction is the product of an infinitesimal function and a bounded function, i.e. function is infinitesimal.

RELATIONSHIP BETWEEN INFINITELY SMALL AND INFINITELY LARGE FUNCTIONS

Theorem 1. If the function f(x) is infinitely large at x→a, then function 1 /f(x) is infinitesimal at x→a.

Proof. Let's take an arbitrary number ε >0 and show that for some δ>0 (depending on ε) for all x, for which |x – a|<δ , the inequality is satisfied, and this will mean that 1/f(x) is an infinitesimal function. Indeed, since f(x) is an infinitely large function at x→a, then there will be δ>0 such that as soon as |x – a|<δ , so | f(x)|> 1/ ε. But then for the same x.

Examples.

The converse theorem can also be proven.

Theorem 2. If the function f(x)- infinitesimal at x→a(or x→∞) and does not vanish, then y= 1/f(x) is an infinitely large function.

Conduct the proof of the theorem yourself.

Examples.

Thus, the simplest properties of infinitesimal and infinitely large functions can be written using the following conditional relations: A≠ 0

LIMIT THEOREMS

Theorem 1. The limit of the algebraic sum of two, three, and generally a certain number of functions is equal to the algebraic sum of the limits of these functions, i.e.

Proof. Let us carry out the proof for two terms, since it can be done in the same way for any number of terms. Let .Then f(x)=b+α(x) And g(x)=c+β(x), Where α And β – infinitesimal functions. Hence,

f(x) + g(x)=(b + c) + (α(x) + β(x)).

Because b+c is a constant and α(x) + β(x) is an infinitesimal function, then

Example. .

Theorem 2. The limit of the product of two, three, and generally a finite number of functions is equal to the product of the limits of these functions:

Proof. Let . Hence, f(x)=b+α(x) And g(x)=c+β(x) And

fg = (b + α)(c + β) = bc + (bβ + cα + αβ).

Work bc there is a constant value. Function bβ + c α + αβ based on the properties of infinitesimal functions, there is an infinitesimal quantity. That's why .

Corollary 1. The constant factor can be taken beyond the limit sign:

.

Corollary 2. The degree limit is equal to the limit degree:

.

Example..

Theorem 3. The limit of the quotient of two functions is equal to the quotient of the limits of these functions if the limit of the denominator is different from zero, i.e.

.

Proof. Let . Hence, f(x)=b+α(x) And g(x)=c+β(x), Where α, β – infinitesimal. Let's consider the quotient

A fraction is an infinitesimal function because the numerator is an infinitesimal function and the denominator has a limit c 2 ≠0.

Examples.

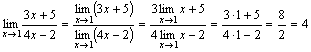
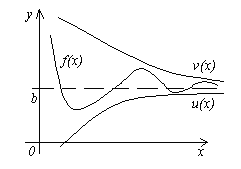
Theorem 4. Let three functions be given f(x), u(x) And v(x), satisfying the inequalities u (x)≤f(x)≤ v(x). If the functions u(x) And v(x) have the same limit at x→a(or x→∞), then the function f(x) tends to the same limit, i.e. If

, That .

The meaning of this theorem is clear from the figure.

The proof of Theorem 4 can be found, for example, in the textbook: Piskunov N. S. Differential and integral calculus, vol. 1 - M.: Nauka, 1985.

Theorem 5. If at x→a(or x→∞) function y=f(x) accepts non-negative values y≥0 and at the same time tends to the limit b, then this limit cannot be negative: b≥0.

Proof. We will carry out the proof by contradiction. Let's pretend that b<0 , Then |y – b|≥|b| and, therefore, the difference modulus does not tend to zero when x→a. But then y doesn't reach the limit b at x→a, which contradicts the conditions of the theorem.

Theorem 6. If two functions f(x) And g(x) for all values ​​of the argument x satisfy the inequality f(x)≥ g(x) and have limits, then the inequality holds b≥c.

Proof. According to the conditions of the theorem f(x)-g(x) ≥0, therefore, by Theorem 5 , or .

UNILATERAL LIMITS

So far we have considered determining the limit of a function when x→a in an arbitrary manner, i.e. the limit of the function did not depend on how it was located x towards a, to the left or right of a. However, it is quite common to find functions that have no limit under this condition, but they do have a limit if x→a, remaining on one side of A, left or right (see figure). Therefore, the concepts of one-sided limits are introduced.

If f(x) tends to the limit b at x tending to a certain number a So x accepts only values ​​less than a, then they write and call blimit of the function f(x) at point a on the left.