Properties of powers in addition. Basic properties of degrees. Examples for multiplying powers with the same exponents
Each arithmetic operation sometimes becomes too cumbersome to write and they try to simplify it. This was once the case with the addition operation. People needed to carry out repeated addition of the same type, for example, to calculate the cost of one hundred Persian carpets, the cost of which is 3 gold coins for each. 3+3+3+…+3 = 300. Due to its cumbersome nature, it was decided to shorten the notation to 3 * 100 = 300. In fact, the notation “three times one hundred” means that you need to take one hundred threes and add them together. Multiplication caught on and gained general popularity. But the world does not stand still, and in the Middle Ages the need arose to carry out repeated multiplication of the same type. I remember an old Indian riddle about a sage who asked for wheat grains in the following quantities as a reward for work done: for the first square of the chessboard he asked for one grain, for the second - two, for the third - four, for the fifth - eight, and so on. This is how the first multiplication of powers appeared, because the number of grains was equal to two to the power of the cell number. For example, on the last cell there would be 2*2*2*...*2 = 2^63 grains, which is equal to a number 18 characters long, which, in fact, is the meaning of the riddle.
The operation of exponentiation caught on quite quickly, and the need to carry out addition, subtraction, division and multiplication of powers also quickly arose. The latter is worth considering in more detail. The formulas for adding powers are simple and easy to remember. In addition, it is very easy to understand where they come from if the power operation is replaced by multiplication. But first you need to understand some basic terminology. The expression a^b (read “a to the power of b”) means that the number a should be multiplied by itself b times, with “a” being called the base of the power, and “b” - power exponent. If the bases of the degrees are the same, then the formulas are derived quite simply. Specific example: find the value of the expression 2^3 * 2^4. To know what should happen, you should find out the answer on the computer before starting the solution. Entering this expression into any online calculator, search engine, typing “multiplying powers with for different reasons and identical" or a mathematical package, the output will be 128. Now let's write out this expression: 2^3 = 2*2*2, and 2^4 = 2*2*2*2. It turns out that 2^3 * 2^4 = 2*2*2*2*2*2*2 = 2^7 = 2^(3+4) It turns out that the product of powers with the same base is equal to the base raised to a power equal to the sum of the two previous powers.
You might think that this is an accident, but no: any other example can only confirm this rule. Thus, in general view the formula looks like this: a^n * a^m = a^(n+m) . There is also a rule that any number to the zero power is equal to one. Here we should remember the rule of negative powers: a^(-n) = 1 / a^n. That is, if 2^3 = 8, then 2^(-3) = 1/8. Using this rule, you can prove the validity of the equality a^0 = 1: a^0 = a^(n-n) = a^n * a^(-n) = a^(n) * 1/a^(n) , a^ (n) can be reduced and one remains. From here the rule is derived that the quotient of powers with the same bases is equal to this base to a degree equal to the quotient of the dividend and divisor: a^n: a^m = a^(n-m) . Example: simplify the expression 2^3 * 2^5 * 2^(-7) *2^0: 2^(-2) . Multiplication is a commutative operation, therefore, you must first add the multiplication exponents: 2^3 * 2^5 * 2^(-7) *2^0 = 2^(3+5-7+0) = 2^1 =2. Next you need to deal with division by negative degree. It is necessary to subtract the exponent of the divisor from the exponent of the dividend: 2^1: 2^(-2) = 2^(1-(-2)) = 2^(1+2) = 2^3 = 8. It turns out that the operation of dividing by a negative the degree is identical to the operation of multiplication by a similar positive exponent. So the final answer is 8.
There are examples where non-canonical multiplication of powers takes place. Multiplying powers with different bases is often much more difficult, and sometimes even impossible. Some examples of different possible techniques should be given. Example: simplify the expression 3^7 * 9^(-2) * 81^3 * 243^(-2) * 729. Obviously, there is a multiplication of powers with different bases. But it should be noted that all bases are different powers of three. 9 = 3^2.1 = 3^4.3 = 3^5.9 = 3^6. Using the rule (a^n) ^m = a^(n*m) , you should rewrite the expression in a more convenient form: 3^7 * (3^2) ^(-2) * (3^4) ^3 * ( 3^5) ^(-2) * 3^6 = 3^7 * 3^(-4) * 3^(12) * 3^(-10) * 3^6 = 3^(7-4+12 -10+6) = 3^(11) . Answer: 3^11. In cases where various grounds, the rule a^n * b^n = (a*b) ^n works for equal indicators. For example, 3^3 * 7^3 = 21^3. Otherwise, when the bases and exponents are different, complete multiplication cannot be performed. Sometimes you can partially simplify or resort to the help of computer technology.
Degree formulas used in the process of reduction and simplification complex expressions, in solving equations and inequalities.
Number c is n-th power of a number a When:
Operations with degrees.
1. By multiplying degrees with the same base, their indicators are added:
a m·a n = a m + n .
2. When dividing degrees with the same base, their exponents are subtracted:

3. Power of the product of 2 or more factors is equal to the product of the powers of these factors:
(abc…) n = a n · b n · c n …
4. The degree of a fraction is equal to the ratio of the degrees of the dividend and the divisor:
(a/b) n = a n /b n .
5. Raising a power to a power, the exponents are multiplied:
(a m) n = a m n .
Each formula above is true in the directions from left to right and vice versa.
For example. (2 3 5/15)² = 2² 3² 5²/15² = 900/225 = 4.
Operations with roots.
1. The root of the product of several factors is equal to the product of the roots of these factors:
2. The root of a ratio is equal to the ratio of the dividend and the divisor of the roots:
![]()
3. When raising a root to a power, it is enough to raise the radical number to this power:

4. If you increase the degree of the root in n once and at the same time build into n th power is a radical number, then the value of the root will not change:
![]()
5. If you reduce the degree of the root in n extract the root at the same time n-th power of a radical number, then the value of the root will not change:

A degree with a negative exponent. The power of a certain number with a non-positive (integer) exponent is defined as one divided by the power of the same number with an exponent equal to absolute value non-positive indicator:

Formula a m:a n =a m - n can be used not only for m> n, but also with m< n.
For example. a4:a 7 = a 4 - 7 = a -3.
To formula a m:a n =a m - n became fair when m=n, the presence of zero degree is required.
A degree with a zero index. The power of any number not equal to zero with a zero exponent is equal to one.
For example. 2 0 = 1,(-5) 0 = 1,(-3/5) 0 = 1.
Degree with a fractional exponent. To raise a real number A to the degree m/n, you need to extract the root n th degree of m-th power of this number A.
Operations with powers and roots. Degree with negative ,
zero and fractional indicator. About expressions that have no meaning.
Operations with degrees.
1. When multiplying powers with the same base, their exponents add up:
a m · a n = a m + n .
2. When dividing degrees with the same base, their exponents are deducted .
3. The degree of the product of two or more factors is equal to the product of the degrees of these factors.
(abc… ) n = a n· b n · c n…
4. The degree of a ratio (fraction) is equal to the ratio of the degrees of the dividend (numerator) and divisor (denominator):
(a/b ) n = a n / b n .
5. When raising a power to a power, their exponents are multiplied:
(a m ) n = a m n .
All the above formulas are read and executed in both directions from left to right and vice versa.
EXAMPLE (2 · 3 · 5 / 15)² = 2² 3² 5² / 15² = 900 / 225 = 4 .
Operations with roots. In all the formulas below, the symbol means arithmetic root (the radical expression is positive).
1. The root of the product of several factors is equal to the product roots of these factors:
2. The root of a ratio is equal to the ratio of the roots of the dividend and the divisor:
![]()
3. When raising a root to a power, it is enough to raise to this power radical number:
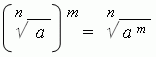
4. If we increase the degree of the root in m raise to m the th power is a radical number, then the value of the root will not change:
5. If we reduce the degree of the root in m extract the root once and at the same time m th power of a radical number, then the value of the root is not will change:

Expanding the concept of degree. So far we have considered degrees only with natural exponents; but actions with degrees and roots can also lead to negative, zero And fractional indicators. All these exponents require additional definition.
A degree with a negative exponent. Power of some number c a negative (integer) exponent is defined as one divided by a power of the same number with an exponent equal to the absolute valuenegative indicator:

T now the formula a m: a n= a m - n can be used not only form, more than n, but also with m, less than n .
EXAMPLE a 4 :a 7 = a 4 - 7 = a - 3 .
If we want the formulaa m : a n= a m - nwas fair whenm = n, we need a definition of degree zero.
A degree with a zero index. The power of any non-zero number with exponent zero is 1.
EXAMPLES. 2 0 = 1, ( – 5) 0 = 1, (– 3 / 5) 0 = 1.
Degree with a fractional exponent. In order to build real number and to the power m/n , you need to extract the root nth power of m -th power of this number A :
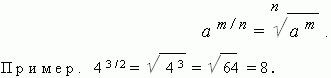
About expressions that have no meaning. There are several such expressions. any number.
In fact, if we assume that this expression is equal to some number x, then according to the definition of the division operation we have: 0 = 0 · x. But this equality occurs when any number x, which was what needed to be proven.
Case 3.
0 0 - any number.
Really,
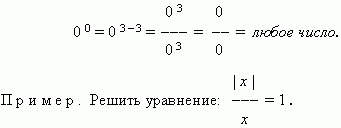
Solution. Let's consider three main cases:
1) x = 0 – this value does not satisfy this equation
(Why?).
2) when x> 0 we get: x/x = 1, i.e. 1 = 1, which means
What x– any number; but taking into account that in
In our case x> 0, the answer isx > 0 ;
3) when x < 0 получаем: – x/x= 1, i.e. e . –1 = 1, therefore,
In this case there is no solution.
Thus, x > 0.
How to multiply powers? Which powers can be multiplied and which cannot? How to multiply a number by a power?
In algebra, you can find a product of powers in two cases:
1) if the degrees have the same bases;
2) if the degrees have the same indicators.
When multiplying powers with the same bases, the base must be left the same, and the exponents must be added:
When multiplying degrees with the same indicators, the overall indicator can be taken out of brackets:
Let's look at how to multiply powers using specific examples.
The unit is not written in the exponent, but when multiplying powers, they take into account:
When multiplying, there can be any number of powers. It should be remembered that you don’t have to write the multiplication sign before the letter:
In expressions, exponentiation is done first.
If you need to multiply a number by a power, you should first perform the exponentiation, and only then the multiplication:
www.algebraclass.ru
Addition, subtraction, multiplication, and division of powers
Addition and subtraction of powers
It is obvious that numbers with powers can be added like other quantities , by adding them one after another with their signs.
So, the sum of a 3 and b 2 is a 3 + b 2.
The sum of a 3 - b n and h 5 -d 4 is a 3 - b n + h 5 - d 4.
Odds equal degrees identical variables can be added or subtracted.
So, the sum of 2a 2 and 3a 2 is equal to 5a 2.
It is also obvious that if you take two squares a, or three squares a, or five squares a.
But degrees various variables And various degrees identical variables, must be composed by adding them with their signs.
So, the sum of a 2 and a 3 is the sum of a 2 + a 3.
It is obvious that the square of a, and the cube of a, is not equal to twice the square of a, but to twice the cube of a.
The sum of a 3 b n and 3a 5 b 6 is a 3 b n + 3a 5 b 6.
Subtraction powers are carried out in the same way as addition, except that the signs of the subtrahends must be changed accordingly.
Or:
2a 4 - (-6a 4) = 8a 4
3h 2 b 6 — 4h 2 b 6 = -h 2 b 6
5(a - h) 6 - 2(a - h) 6 = 3(a - h) 6
Multiplying powers
Numbers with powers can be multiplied, like other quantities, by writing them one after the other, with or without a multiplication sign between them.
Thus, the result of multiplying a 3 by b 2 is a 3 b 2 or aaabb.
Or:
x -3 ⋅ a m = a m x -3
3a 6 y 2 ⋅ (-2x) = -6a 6 xy 2
a 2 b 3 y 2 ⋅ a 3 b 2 y = a 2 b 3 y 2 a 3 b 2 y
The result in the last example can be ordered by adding identical variables.
The expression will take the form: a 5 b 5 y 3.
By comparing several numbers (variables) with powers, we can see that if any two of them are multiplied, then the result is a number (variable) with a power equal to amount degrees of terms.
So, a 2 .a 3 = aa.aaa = aaaaa = a 5 .
Here 5 is the power of the multiplication result, which is equal to 2 + 3, the sum of the powers of the terms.
So, a n .a m = a m+n .
For a n , a is taken as a factor as many times as the power of n;
And a m is taken as a factor as many times as the degree m is equal to;
That's why, powers with the same bases can be multiplied by adding the exponents of the powers.
So, a 2 .a 6 = a 2+6 = a 8 . And x 3 .x 2 .x = x 3+2+1 = x 6 .
Or:
4a n ⋅ 2a n = 8a 2n
b 2 y 3 ⋅ b 4 y = b 6 y 4
(b + h - y) n ⋅ (b + h - y) = (b + h - y) n+1
Multiply (x 3 + x 2 y + xy 2 + y 3) ⋅ (x - y).
Answer: x 4 - y 4.
Multiply (x 3 + x – 5) ⋅ (2x 3 + x + 1).
This rule is also true for numbers whose exponents are negative.
1. So, a -2 .a -3 = a -5 . This can be written as (1/aa).(1/aaa) = 1/aaaaa.
2. y -n .y -m = y -n-m .
3. a -n .a m = a m-n .
If a + b are multiplied by a - b, the result will be a 2 - b 2: that is
The result of multiplying the sum or difference of two numbers equal to the sum or the difference of their squares.
If you multiply the sum and difference of two numbers raised to square, the result will be equal to the sum or difference of these numbers in fourth degrees.
So, (a - y).(a + y) = a 2 - y 2.
(a 2 - y 2)⋅(a 2 + y 2) = a 4 - y 4.
(a 4 - y 4)⋅(a 4 + y 4) = a 8 - y 8.
Division of degrees
Numbers with powers can be divided like other numbers, by subtracting from the dividend, or by placing them in fraction form.
Thus, a 3 b 2 divided by b 2 is equal to a 3.
Writing a 5 divided by a 3 looks like $\frac $. But this is equal to a 2 . In a series of numbers
a +4 , a +3 , a +2 , a +1 , a 0 , a -1 , a -2 , a -3 , a -4 .
any number can be divided by another, and the exponent will be equal to difference indicators of divisible numbers.
When dividing degrees with the same base, their exponents are subtracted..
So, y 3:y 2 = y 3-2 = y 1. That is, $\frac = y$.
And a n+1:a = a n+1-1 = a n . That is, $\frac = a^n$.
Or:
y 2m: y m = y m
8a n+m: 4a m = 2a n
12(b + y) n: 3(b + y) 3 = 4(b +y) n-3
The rule is also true for numbers with negative values of degrees.
The result of dividing a -5 by a -3 is a -2.
Also, $\frac: \frac = \frac .\frac = \frac = \frac $.
h 2:h -1 = h 2+1 = h 3 or $h^2:\frac = h^2.\frac = h^3$
It is necessary to master multiplication and division of powers very well, since such operations are very widely used in algebra.
Examples of solving examples with fractions containing numbers with powers
1. Decrease the exponents by $\frac $ Answer: $\frac $.
2. Decrease exponents by $\frac$. Answer: $\frac$ or 2x.
3. Reduce the exponents a 2 /a 3 and a -3 /a -4 and bring to a common denominator.
a 2 .a -4 is a -2 the first numerator.
a 3 .a -3 is a 0 = 1, the second numerator.
a 3 .a -4 is a -1 , the common numerator.
After simplification: a -2 /a -1 and 1/a -1 .
4. Reduce the exponents 2a 4 /5a 3 and 2 /a 4 and bring to a common denominator.
Answer: 2a 3 /5a 7 and 5a 5 /5a 7 or 2a 3 /5a 2 and 5/5a 2.
5. Multiply (a 3 + b)/b 4 by (a - b)/3.
6. Multiply (a 5 + 1)/x 2 by (b 2 - 1)/(x + a).
7. Multiply b 4 /a -2 by h -3 /x and a n /y -3 .
8. Divide a 4 /y 3 by a 3 /y 2 . Answer: a/y.
Properties of degree
We remind you that in this lesson we will understand properties of degrees with natural indicators and zero. Degrees with rational indicators and their properties will be discussed in lessons for 8th grade.
A power with a natural exponent has several important properties that allow us to simplify calculations in examples with powers.
Property No. 1
Product of powers
When multiplying powers with the same bases, the base remains unchanged, and the exponents of the powers are added.
a m · a n = a m + n, where “a” is any number, and “m”, “n” are any natural numbers.
This property of degrees also applies to product of three and more degrees.
b b 2 b 3 b 4 b 5 = b 1 + 2 + 3 + 4 + 5 = b 15
6 15 36 = 6 15 6 2 = 6 15 6 2 = 6 17
(0.8) 3 · (0.8) 12 = (0.8) 3 + 12 = (0.8) 15
Please note that in the specified property we were talking only about the multiplication of powers with the same bases. It does not apply to their addition.
You cannot replace the sum (3 3 + 3 2) with 3 5. This is understandable if
calculate (3 3 + 3 2) = (27 + 9) = 36, and 3 5 = 243
Property No. 2
Partial degrees
When dividing powers with the same bases, the base remains unchanged, and the exponent of the divisor is subtracted from the exponent of the dividend.
(2b) 5: (2b) 3 = (2b) 5 − 3 = (2b) 2
11 3 − 2 4 2 − 1 = 11 4 = 44
Example. Solve the equation. We use the property of quotient powers.
3 8: t = 3 4
Answer: t = 3 4 = 81
Using properties No. 1 and No. 2, you can easily simplify expressions and perform calculations.
- Example. Simplify the expression.
4 5m + 6 4 m + 2: 4 4m + 3 = 4 5m + 6 + m + 2: 4 4m + 3 = 4 6m + 8 − 4m − 3 = 4 2m + 5
Example. Find the value of an expression using the properties of exponents.
2 11 − 5 = 2 6 = 64
Please note that in Property 2 we were only talking about dividing powers with the same bases.
You cannot replace the difference (4 3 −4 2) with 4 1. This is understandable if you calculate (4 3 −4 2) = (64 − 16) = 48, and 4 1 = 4
Property No. 3
Raising a degree to a power
When raising a degree to a power, the base of the degree remains unchanged, and the exponents are multiplied.
(a n) m = a n · m, where “a” is any number, and “m”, “n” are any natural numbers.

Please note that property No. 4, like other properties of degrees, is also applied in reverse order.
(a n b n)= (a b) n
That is, to multiply powers with the same exponents, you can multiply the bases, but leave the exponent unchanged.
2 4 5 4 = (2 5) 4 = 10 4 = 10,000
0.5 16 2 16 = (0.5 2) 16 = 1
In more complex examples There may be cases when multiplication and division must be performed over powers with different bases and different indicators. In this case, we advise you to do the following.
For example, 4 5 3 2 = 4 3 4 2 3 2 = 4 3 (4 3) 2 = 64 12 2 = 64 144 = 9216
An example of raising a decimal to a power.
4 21 (−0.25) 20 = 4 4 20 (−0.25) 20 = 4 (4 (−0.25)) 20 = 4 (−1) 20 = 4 1 = 4
Properties 5
Power of a quotient (fraction)
To raise a quotient to a power, you can raise the dividend and the divisor separately to this power, and divide the first result by the second.
(a: b) n = a n: b n, where “a”, “b” are any rational numbers, b ≠ 0, n - any natural number.
(5: 3) 12 = 5 12: 3 12
We remind you that a quotient can be represented as a fraction. Therefore, we will dwell on the topic of raising a fraction to a power in more detail on the next page.
Powers and roots
Operations with powers and roots. Degree with negative ,
zero and fractional indicator. About expressions that have no meaning.
Operations with degrees.
1. When multiplying powers with the same base, their exponents are added:
a m · a n = a m + n .
2. When dividing degrees with the same base, their exponents are deducted .

3. The degree of the product of two or more factors is equal to the product of the degrees of these factors.
4. The degree of a ratio (fraction) is equal to the ratio of the degrees of the dividend (numerator) and divisor (denominator):
(a/b) n = a n / b n .
5. When raising a power to a power, their exponents are multiplied:
All the above formulas are read and executed in both directions from left to right and vice versa.
EXAMPLE (2 3 5 / 15)² = 2² · 3² · 5² / 15² = 900 / 225 = 4 .
Operations with roots. In all the formulas below, the symbol means arithmetic root(the radical expression is positive).
1. The root of the product of several factors is equal to the product of the roots of these factors:
2. The root of a ratio is equal to the ratio of the roots of the dividend and the divisor:
![]()
3. When raising a root to a power, it is enough to raise to this power radical number:
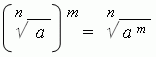
4. If you increase the degree of the root by m times and at the same time raise the radical number to the mth power, then the value of the root will not change:
![]()
5. If you reduce the degree of the root by m times and simultaneously extract the mth root of the radical number, then the value of the root will not change:
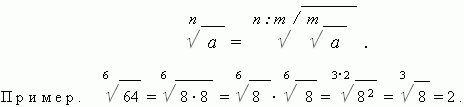
Expanding the concept of degree. So far we have considered degrees only with natural exponents; but operations with powers and roots can also lead to negative, zero And fractional indicators. All these exponents require additional definition.
A degree with a negative exponent. The power of a certain number with a negative (integer) exponent is defined as one divided by the power of the same number with an exponent equal to the absolute value of the negative exponent:

Now the formula a m : a n = a m - n can be used not only for m, more than n, but also with m, less than n .
EXAMPLE a 4: a 7 = a 4 — 7 = a — 3 .
If we want the formula a m : a n = a m — n was fair when m = n, we need a definition of degree zero.
A degree with a zero index. The power of any non-zero number with exponent zero is 1.
EXAMPLES. 2 0 = 1, ( – 5) 0 = 1, (– 3 / 5) 0 = 1.
Degree with a fractional exponent. In order to raise a real number a to the power m / n, you need to extract the nth root of the mth power of this number a:

About expressions that have no meaning. There are several such expressions.
Where a ≠ 0 , does not exist.
In fact, if we assume that x is a certain number, then in accordance with the definition of the division operation we have: a = 0· x, i.e. a= 0, which contradicts the condition: a ≠ 0
— any number.
In fact, if we assume that this expression is equal to some number x, then according to the definition of the division operation we have: 0 = 0 · x. But this equality occurs when any number x, which was what needed to be proven.
0 0 — any number.

Solution. Let's consider three main cases:
1) x = 0 – this value does not satisfy this equation
2) when x> 0 we get: x/x= 1, i.e. 1 = 1, which means
What x– any number; but taking into account that in
in our case x> 0, the answer is x > 0 ;
Rules for multiplying powers with different bases
DEGREE WITH RATIONAL INDICATOR,
POWER FUNCTION IV
§ 69. Multiplication and division of powers with the same bases
Theorem 1. To multiply powers with the same bases, it is enough to add the exponents and leave the base the same, that is
Proof. By definition of degree
2 2 2 3 = 2 5 = 32; (-3) (-3) 3 = (-3) 4 = 81.
We looked at the product of two powers. In fact, the proven property is true for any number of powers with the same bases.
Theorem 2. To divide powers with the same bases, when the index of the dividend is greater than the index of the divisor, it is enough to subtract the index of the divisor from the index of the dividend, and leave the base the same, that is at t > p
(a =/= 0)
Proof. Recall that the quotient of dividing one number by another is the number that, when multiplied by the divisor, gives the dividend. Therefore, prove the formula where a =/= 0, it's the same as proving the formula
If t > p , then the number t - p will be natural; therefore, by Theorem 1
Theorem 2 is proven.
It should be noted that the formula
we have proved it only under the assumption that t > p . Therefore, from what has been proven, it is not yet possible to draw, for example, the following conclusions:
![]()
In addition, we have not yet considered degrees with negative exponents and we do not yet know what meaning can be given to expression 3 - 2 .
Theorem 3. To raise a degree to a power, it is enough to multiply the exponents, leaving the base of the degree the same, that is
Proof. Using the definition of degree and Theorem 1 of this section, we obtain:

Q.E.D.
For example, (2 3) 2 = 2 6 = 64;
![]()
518 (Oral) Determine X from the equations:
1) 2 2 2 2 3 2 4 2 5 2 6 = 2 x ; 3) 4 2 4 4 4 6 4 8 4 10 = 2 x ;
2) 3 3 3 3 5 3 7 3 9 = 3 x ; 4) 1 / 5 1 / 25 1 / 125 1 / 625 = 1 / 5 x .
519. (Set no.) Simplify:

520. (Set no.) Simplify:
521. Present these expressions in the form of degrees with the same bases:
1) 32 and 64; 3) 8 5 and 16 3; 5) 4 100 and 32 50;
2) -1000 and 100; 4) -27 and -243; 6) 81 75 8 200 and 3 600 4 150.
